What Would It Take to Move Mercury?

_{[Considerable technical content this month. For those unfamiliar with the subject,}_{I recommend Robert Braeunig’s introduction to basic orbital mechanics.]}

When it comes to space colonization, Mars gets all the love. This makes a certain amount of sense—it’s the most similar planet to Earth and the easiest target for crewed missions. NASA spends a lot of time talking about its #JourneyToMars and Elon Musk explicitly wants to transport humans to the red planet. Many believe that we should terraform Mars to make it even closer to terrestrial conditions (SpaceX currently has concept art of this process as its cover photo on Twitter). More ~~rational~~ radical thinkers will argue that free-floating habitats are more efficient than surface-bound cities, but we’re a long way from developing sustainable, closed-cycle life support systems.

After Mars, however, there’s a lot of options for spreading humanity through the Solar System. Resource extraction could drive colonization of the Asteroid Belt, and there’s plenty of available real estate on Luna and the moons of the outer planets. Ambitious planners propose terraforming Venus, converting the atmosphere to breathable oxygen (though shortening the day to a reasonable length remains a challenging problem).

The viability of terraforming depends in no small part on the body’s distance from the Sun, and one terrestrial planet is almost certainly too close: Mercury. Since at least high school, I’ve been wondering if we could move it to a more suitable orbit. Now that I know I thing or two about orbital mechanics and space transportation, let’s try answering that question.

Enhanced-color image of Mercury taken by the MESSENGER spacecraft.

Source: NASA/Johns Hopkins University Applied Physics Laboratory/Carnegie Institution of Washington

First of all, we need to select Mercury’s destination orbit. For the purposes of these calculations, the destination will be a circular orbit at 1.25 astronomical units, with the same inclination as Earth. I chose this orbit because it’s the furthest possible from other planets in the habitable zone (to a nice round number, at least). We’ll be neglecting any gravitational interactions between planets in this post, but those effects could be significant in a higher-fidelity model. Timing will probably be important.

We can use the Vis-Viva Equation to calculate the transfer between Mercury’s orbit and a circular, 1.25-AU orbit:

$V = \sqrt{\dfrac{2\mu}{r}-\dfrac{\mu}{a}}$

where $V$ is the orbiting body’s velocity at a given point in time, $\mu$ is the standard gravitational parameter of the body being orbited (in this case, Sol), $r$ is the current distance of the orbiting body from the primary, and $a$ is the semi-major axis of the orbital ellipse.

We can use the Vis-Viva Equation to calculate the velocity difference or $\Delta V$ between Mercury on its current orbit and its velocity at the start of the transfer orbit. The transfer should occur at perihelion to exploit the Oberth effect. Mercury has an unusually elliptical orbit, so this won’t be a trivial factor.

We can look up Mercury’s perihelion $r_p$ and semi-major axis $a_m$ in a table; the transfer orbit’s semi-major axis $a_t$ we’ll have to calculate:

$a_t = \frac{r_p + r_f}{2} = \frac{0.3075 + 1.25 \textrm{AU}}{2} = \frac{46.00\times 10^6\ \textrm{km} + 186.5\times 10^6\ \textrm{km}}{2} = 116.3\ \textrm{million km}$

Plugging these values into the Vis-Viva Equation, we can calculate the $\Delta V$ for the outbound transfer:

$\Delta V_1= V_{p,t} - V_{p,m} = \sqrt{\frac{2\mu_\odot}{r_p} - \frac{\mu_\odot}{a_m}} - \sqrt{\frac{2\mu_\odot}{r_p} - \frac{\mu_\odot}{a_t}} = 9.0558\ \textrm{km/s}$

We can perform a similar calculation to find the $\Delta V$ for the circularization maneuver at the apoapsis $r_f$ of the transfer orbit:

$\Delta V_3 = V_{f} - V_{a,t} = \sqrt{\frac{\mu_\odot}{r_f}} - \sqrt{\frac{2\mu_\odot}{r_f} - \frac{\mu_\odot}{a_t}} = 9.8953\ \textrm{km/s}$

You may have noticed that we skipped from $\Delta V_1$ to $\Delta V_3$ . This is because Mercury has a highly-inclined orbit relative to the rest of the inner planets. To correct this, we will need to perform some sort of plane-change maneuver $\Delta V_2$ .

Plane change maneuvers are slightly more complicated to compute; they involve the body’s velocity at the point of the maneuver $V_i$ and the inclination angle $\alpha$ between the two planes. The $\Delta V$ for the maneuver is given by the equation:

$\Delta V_2 = 2V_i \sin\bigg(\dfrac{\alpha}{2}\bigg)$

The inclination between Mercury and Earth is a known value; the location (and therefore, velocity) of the plane change is a design choice. A few mathematical guidelines inform our decision:

Plane changes should occur as far away from the primary body as possible, to minimize $V_i$ per the Vis-Viva Equation.
A normal $\Delta V$ can complete the maneuver only at the ascending or descending node between the two orbits.
Non-normal impulses will tend to modify the orbit from the transfer ellipse calculated above.

To compute the plane change, then, we would like to determine the location of the ascending or descending node. Theoretically, we could do the plane change more efficiently through maneuvers near aphelion, but I’ve already spend enough time on minutiae for what I’d originally intended as a back-of-the-envelope calculation.

Node angles are the sort of thing we look up in a table; I’ve kept Brown and Vallado on hand throughout this project.¹ Mercury’s longitude of the ascending node $\Omega_\textrm{AN}$ is at 48.318° (compared to the First Point of Aries), while its longitude of perihelion $\overline{\omega}$ occurs at 77.438°. This tells us that Mercury’s ascending node lies before perihelion—in other words, the transfer orbit will pass through the descending node on its way to the destination orbit, not the ascending node.

The longitude of the descending node will be 180° ahead of the longitude of the ascending node, therefore $\Omega_{\textrm{DN}} = 228.318\textrm{\textdegree}$ . Subtracting the longitude of perihelion, we can find the true anomaly $\nu$ of the transfer orbit at the descending node:

$\nu_\textrm{DN} = \Omega_\textrm{DN} - \overline{\omega} = 228.218\textrm{\textdegree} - 77.438\textrm{\textdegree} = 150.880\textrm{\textdegree}$

This value in hand, we can compute the distance $r_\textrm{DN}$ of Mercury from the Sun at that point along the transfer orbit:

$r_\textrm{DN} = \dfrac{a_t (1 - e_t^2)}{1 + e_t \cos(\nu_\textrm{DN})}$

where $e_t$ is the eccentricity of the transfer orbit:

$e_t = 1 - \dfrac{r_p}{a_t} = 1 - \dfrac{46.00\times 10^6\ \textrm{km}}{116.3\times 10^6\ \textrm{km}} = 0.6043$

From these values, we find that $r_\textrm{DN}$ occurs at 156.3 million km, about the distance of Earth from the Sun. Plugging this into the Vis-Viva Equation, we find that Mercury will be moving 23.5622 km/s when it passes the descending node. Consequently:

$\Delta V_2 = 2\ (23.5856\ \textrm{km/s}) \times \sin\bigg(\dfrac{7.005 \textrm{\textdegree}}{2}\bigg) = 2.8818\ \textrm{km/s}$

Thus the total $\Delta V$ requirement is:

$\Sigma\Delta V = \Delta V_1 + \Delta V_2 + \Delta V_3 =\ 21.8329\ \textrm{km/s}$

This result tells us what we already knew: getting back to Earth from Mercury is no mean feat. It’s going to be awhile before we see sample return missions, let alone crewed expeditions. Larger missions are more difficult to achieve, because they require more energy. From the Newtonian² definition of kinetic energy $\textrm{KE}$ :

$\textrm{KE} = \frac{1}{2}mV^2$

where $m$ is the mass of the body in question and $V$ is the velocity of that body. Imparting a larger change in velocity, or imparting a velocity change to a more massive body, requires more energy. This is why uncrewed probes are easier to launch than crewed missions (human beings require all this pesky “elbow room” and “life support” that robots can do without).

When we look at moving planets the numbers become absolutely, well, astronomical. The mass of Mercury is about $3.3022\times 10^{23}\ \textrm{kg}$ . When we plug this value and the initial and final velocities of each maneuver into the kinetic energy equation, we can compute the total kinetic energy $\Sigma\Delta\textrm{KE}$ required for the transfer:

$\Sigma\Delta\textrm{KE} = \Delta\textrm{KE}_1 + \Delta\textrm{KE}_2 + \Delta\textrm{KE}_3$

$= 1.8990 \times 10^{32}\ \textrm{J} + 1.3712 \times 10^{30}\ \textrm{J} + 7.0998 \times 10^{31}\ \textrm{J}$

$\therefore \Sigma\Delta\textrm{KE} = 2.6227 \times 10^{32}\ \textrm{J}$

This is a ridiculously large number. The metric prefix system doesn’t even go that high. At this point I just have to trust my calculations, rather than rely on any intuition about orbital mechanics.³

Let’s compare this number to other energy values to get a sense for just what sort of technology we’ll need to move Mercury to a more suitable orbit.

For a long time, my thought was that we could achieve this project by liquidating Earth’s nuclear stockpile. However, the Hiroshima Bomb released only 63 terajoules of energy. The Tsar Bomba, the largest nuclear weapon ever tested, released 210 petajoules. Moving Mercury, by my calculations, would require 4.16 quintillion Hiroshima Bombs, or 1.25 quadrillion Tsar Bombae.⁴

The remaining global nuclear arsenal comes nowhere close to that size, and remember that we’re assuming a perfect conversion of released energy into acceleration. In practice, the efficiency of nuclear pulse propulsion would probably be much closer to 10% than 100%.

Let’s ignore that question for now and focus on something more important: do we have access to that quantity of energy at all?

According to BP, global energy consumption in 2017 was about 13,500 million tons of oil equivalent (Mtoe), an unusual unit that I had never heard of before this project. Fortunately, the International Energy Agency provides a converter on their website, which informs us that the annual energy consumption of human civilization is about 565.218 exa-joules. Moving Mercury will therefore require 464 trillion times the current energy consumption of human civilization.

That figure is still small compared to the energy output of the Sun. Scientists estimate Sol releases 384.6 yotta-joules per second, or $12.129 \times 10^{33}\ \textrm{J/yr}$ . Thus, moving Mercury would require only about 2.2% of the Sun’s annual energy output.

The exact $\Delta V$ requirement of an interplanetary transfer necessarily depends on the precise destination orbit. Maintaining all the trajectory assumptions above, we can compare the energy requirements to move Mercury to different distances from the Sun.

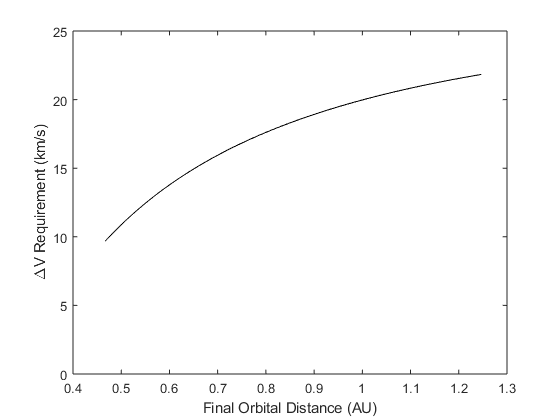
These values apply to any such transfer between Mercury’s perihelion distance and a circular, Earth-coplanar destination orbit.

These values apply only for an object with the approximate mass of Mercury.

Because I could, I decided to prepare plots expressing the energy requirement in terms of Hiroshima bombs and Tsar Bombae. These don’t really add any value besides reaffirming the extreme forces necessary for astronomical engineering projects, but they are amusing.

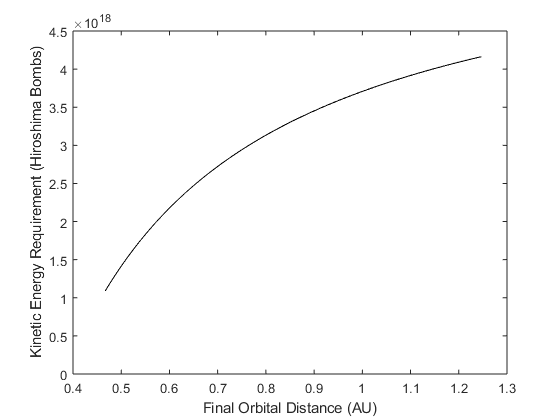
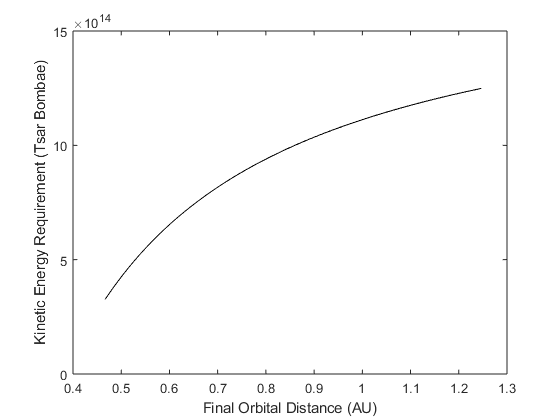
These maintain the assumption that Mercury’s final orbit will be coplanar with Earth’s; however, plane change maneuvers are often an extremely expensive part of a spacecraft’s mission profile. If we eliminate $\Delta V_2$ we can shave several kilometers per second off the total. Conversely, performing a larger plane change could dramatically increase the mission requirements.

I wanted to see how the inclination change interacted with final orbital distance to affect the energy requirements. I calculated the $\Delta V$ to move Mercury over the range of destination distances above, with inclination increments of 1°. Note that performing an inclination change of greater than 90° is equivalent to performing a smaller change in the opposite direction.

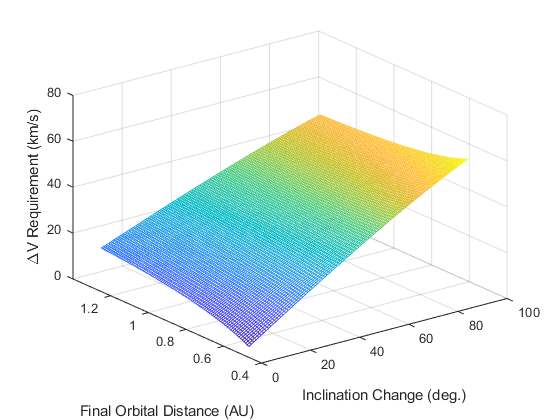
These values apply to any transfer between Mercury’s perihelion distance and a circular destination orbit.

These values apply only to an object with the approximate mass of Mercury.

As you can see, inclination changes significantly increase the $\Delta V$ and energy needed to perform the transfer, particularly at high inclinations for close orbits. It’s unlikely that anyone would want to put Mercury into an orbit well off the ecliptic, but it’s certainly conceivable. When we have this kind of energy at our disposal, all sorts of possibilities open up.

When will we have that amount of energy available? It’s hard to say, but not for a really long time—sufficiently long that many of the problems which moving Mercury could address probably wouldn’t matter anymore. For instance, putting a large body in orbit of Mars or Venus could jumpstart their magnetic fields, and in the latter case decrease the rotational period to something more Earth-like. If we have yotta-joules on tap, however, we probably wouldn’t need such crude solutions.⁵

Simply put, moving planets is the domain of Type II civilizations, because it requires energies on the order of stellar outputs. Carl Sagan proposed an equation to estimate Kardashev levels, as follows:

$K = \dfrac{\log_{10}P - 6}{10}$

where $K$ is the civilization’s Kardashev level and $P$ is the civilization’s average power consumption in Watts. We can find $P$ by converting the energy usage to Watt-hours, and then dividing by the 8760 hours in a year. Using the results of my calculations, $P = 8.317\ \textrm{YW}$ and therefore $K = 1.89$ . In other words, moving Mercury to 1.25 AU would consume as much energy as an almost-Type II civilization would need for an entire year.

I think it’s safe to say that conventional rocket or pulse propulsion systems will not be up for this task.⁶ Continuous-thrust propulsion systems, on the other hand, might make the energy accounting more tractable by amortizing the requirement over years, decades, or even centuries. Megascale solar sails would be an attractive option because they take energy directly from the Sun, rather than other sources. After the initial production investment, solar sails would require relatively little additional energy input from a civilization’s reserves (compared to other propulsion options).

As I learned working with ion thrusters in my senior capstone, continuous-thrust propulsion can be a lot more challenging to model than impulsive systems. Spiraling Mercury outward would entail much more involved timing to ensure planetary interaction risks are minimal. Given that society becomes more risk averse with increased resources, a civilization capable of moving planets might choose not to.

Quite frankly, I wouldn’t blame them. As we’ve seen, moving planets is an expensive proposition, and there’s a lot of other things that civilization could be spending those resources on. Someday, we may have enough energy available that even astronomical engineering seems trivial, but for now colonizing Mars is a much more important project.

References

“BP Statistical Review of World Energy”, 67th ed., BP plc, London, UK, June 2018.
Brown, C. D., Elements of Spacecraft Design, edited by J. S. Przemieniecki, AIAA Education Series, AIAA, Reston VA, 2003.
Grayzeck, E. J. , “Mercury Fact Sheet”, NASA Space Science Data Coordinated Archive, 27 Sept. 2018, https://nssdc.gsfc.nasa.gov/planetary/factsheet/mercuryfact.html.
Vallado, D. A., Fundamentals of Astrodynamics and Applications, 4th ed., Microcosm, Hawthorne CA, 2013.

¹The values in these tables are adequate for preliminary mission design, but are subject to change over the long term. In general practice, it’s worth using updated data for higher accuracy.

²The Newtonian formulation mostly applies, i.e. the Lorenz factor $\gamma$ is small. Note that relativistic effects do apply to Mercury’s orbit; I’m choosing to neglect them for these preliminary estimates. Serious astronomical engineers should take those into account.

³I am reasonably confident in my calculations, or I wouldn’t be writing about them publicly. That said, if I made a mistake, then kinetic energy required for the plane change is probably where.

⁴Bombae is my preferred pluralization of Bomba and I will accept no substitutes.

⁵Shortening Venus’s day might take less energy than moving Mercury, but that will have to be a different post.

⁶Assuming a rocket engine with an exhaust velocity of c, we would need about $2.1\times 10^{19}\ \textrm{kg}$ of reaction mass to change Mercury’s orbit. This is about the same mass as the asteroid 3 Juno, or about 1% the total mass of the Asteroid Belt.

Nathaniel M. Routh

It's More Like Rocket Engineering

What Would It Take to Move Mercury?

References

Leave a comment Cancel reply